How to Subtract Thousands

MediaScope

Editor

posted on 10 years ago — updated on 1 second ago

21
views

As you begin to subtract bigger and bigger numbers, the rules of subtraction stay the same; however, the calculations can become trickier because often you will need to regroup, or borrow, several times. Borrowing is when you borrow from a larger place value so that you can subtract a larger digit from a smaller digit. As long as you align your numbers neatly, and keep track of all digits as they change through regrouping, subtracting thousands (and other 4-digit numbers), is no more difficult than subtracting smaller numbers.

Steps

Subtracting a Smaller Number from a Larger Number

Identify the larger number. Generally when subtracting, you subtract the smaller number from the larger number, which results in a positive answer, or difference.

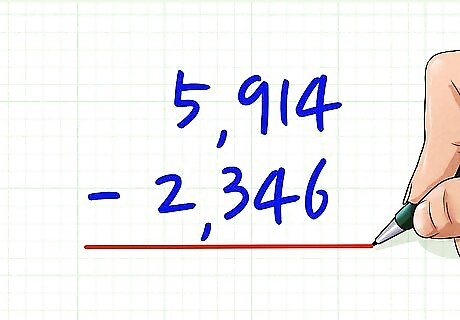
Line up the problems vertically. The larger number should be on the top. Ensure the digits in each place value are lined up; that is, the ones place in the top number should be lined up vertically with the ones place in the bottom number, the tens place in the top should be lined up with the tens place in the bottom, etc. The place values in a 4-digit number, from right-to-left, are the ones place, tens place, hundreds place, and thousands place. The place values should always be aligned. Even if you are subtracting a 4-digit number from a 1-digit number. Since a 1-digit number only has ones, you will align this number with the digit in the ones place of the larger number.

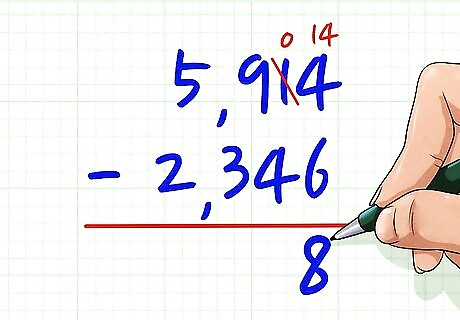
Subtract the digits in the ones place. Remember, the ones place is on the far right. If the bottom digit is larger than the top digit, you must borrow a ten from the tens place. To do this, subtract 1 from the digit in the tens place, and add 10 to the digit in the ones place. Make sure you cross out the original digits and write the new numbers after borrowing. For example, if you are calculating 5 , 914 − 2 , 346 {\displaystyle 5,914-2,346} 5,914-2,346, you will subtract 4 − 6 {\displaystyle 4-6} 4-6 in the ones place. Since 6 is larger than 4, you must borrow from the tens place. The 1 in the tens place becomes a 0, and the 4 in the ones place becomes 14. So now you will calculate 14 − 6 = 8 {\displaystyle 14-6=8} 14-6=8. So the digit in the ones place of your answer is 8.

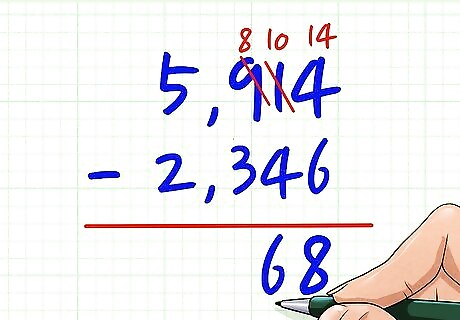
Subtract the digits in the tens place. The tens place is the digit second from the right. Remember to use the new digit if you borrowed from the tens place when subtracting the ones place. If the bottom digit is larger than the top digit, you will have to borrow from the hundreds place. To borrow from the hundreds place, follow the same procedure you used when borrowing from the tens place. For example, if you are calculating 5 , 914 − 2 , 346 {\displaystyle 5,914-2,346} 5,914-2,346, you will subtract 0 − 4 {\displaystyle 0-4} 0-4 in the tens place, since you borrowed a ten when subtracting ones. Since 4 is larger than 0, you must borrow from the hundreds place. The 9 in the hundreds place becomes an 8 , and the 0 in the tens place becomes 10. So now you will calculate 10 − 4 = 6 {\displaystyle 10-4=6} 10-4=6. So the digit in the tens place of your answer is 6.

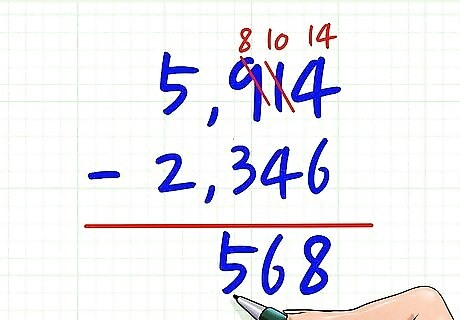
Subtract the digits in the hundreds place. The hundreds place is the digit third from the right. Remember to use the new digit if you borrowed previously, and to borrow from the thousands place, using the same borrowing procedure, if the bottom digit is larger than the top digit. For example, if you are calculating 5 , 914 − 2 , 346 {\displaystyle 5,914-2,346} 5,914-2,346, you will subtract 8 − 3 {\displaystyle 8-3} 8-3 in the hundreds place, since you borrowed a hundred when subtracting tens. 8 − 3 = 5 {\displaystyle 8-3=5} 8-3=5. So the digit in the hundreds place of your answer is 5.

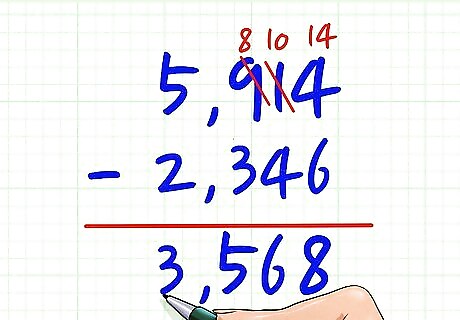
Subtract the digits in the thousands place. The thousands place is the digit on the far left. Make sure you use any new digits after borrowing. You will not have to borrow in the thousands place, since the top number is ultimately larger than the bottom number. For example, if you are calculating 5 , 914 − 2 , 346 {\displaystyle 5,914-2,346} 5,914-2,346, you will subtract 5 − 2 {\displaystyle 5-2} 5-2 in the thousands place. 5 − 2 = 3 {\displaystyle 5-2=3} 5-2=3. So, 5 , 914 − 2 , 346 = 3 , 568 {\displaystyle 5,914-2,346=3,568} 5,914-2,346=3,568.

Check your answer. Since it is easy to make mistakes when subtracting larger numbers, it is always a good idea to check your answer with a calculator.

Subtracting a Larger Number from a Smaller Number

Identify the larger number. Usually in subtraction, you will subtract a smaller number from a larger number. Sometimes, however, you will be asked to subtract a larger number from a smaller number. In this instance, the answer, or difference, will be negative. For example, if you are subtracting 2 , 346 − 5 , 914 {\displaystyle 2,346-5,914} 2,346-5,914, you should note that 5,914 is larger. Since you are subtracting a larger number from a smaller number, your answer will be negative.

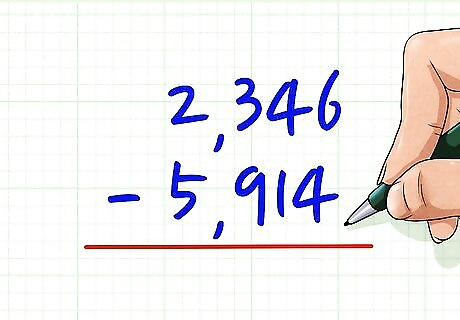
Line up the problems vertically. Even though you are supposed to subtract the larger number from the smaller, for this method you need to place the larger number on top. Ensure the digits in each place value are lined up. The place values in a 4-digit whole number, from right-to-left, are the ones place, tens place, hundreds place, and thousands place. For example, if you are subtracting 2 , 346 − 5 , 914 {\displaystyle 2,346-5,914} 2,346-5,914, even though you are supposed to take away 5,914, you will set up the problem with 5,914 on top.

Subtract the digits in each place value. You will need to borrow from other place values if the digit on top is smaller than the digit on the bottom. For example, after borrowing to subtract the digits in each place value, you should calculate 5 , 914 − 2 , 346 = 3 , 568 {\displaystyle 5,914-2,346=3,568} 5,914-2,346=3,568.

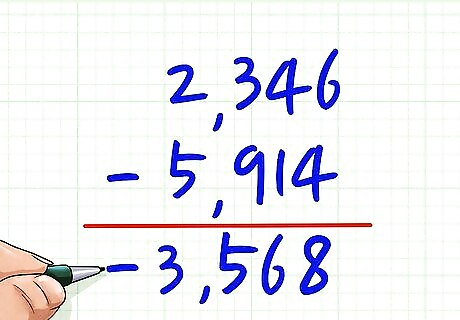
Place a negative sign in front of your answer. The difference between two numbers is always the same, regardless of how you subtract them. Remember, however, that when subtracting a larger number from a smaller number, as the original problem stated, the answer will result in a negative number. For example, if 5 , 914 − 2 , 346 = 3 , 568 {\displaystyle 5,914-2,346=3,568} 5,914-2,346=3,568, then 2 , 346 − 5 , 914 = − 3 , 568 {\displaystyle 2,346-5,914=-3,568} 2,346-5,914=-3,568.

Check your answer. It is easy to make mistakes when you need to borrow to subtract, so it’s a good idea to check your answer with a calculator.

Subtracting Numbers with Decimals

Line up the problems vertically. The number you are subtracting from should be on top, and the decimal points should be aligned, as well as the digits in each place value. Fill any blank spaces in front of a decimal with zeros. For whole numbers, place a decimal point to the right of the ones place, and fill any blank space behind the number with zeros. For example, if you are subtracting 4 , 750 − 136.2 {\displaystyle 4,750-136.2} 4,750-136.2, you would place a decimal after the 0 in 4,750, and set up the problem with 4,750.0 on top of 0136.2.

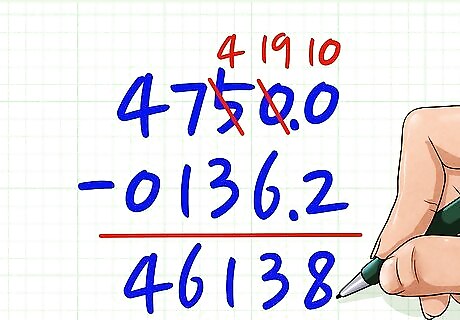
Subtract the digits in each place value. Ignore the decimal points for now, and subtract as if you were working with two whole numbers. You will need to borrow from other place values if the digit on top is smaller than the digit on the bottom. For example, after borrowing to subtract the digits in each place value, you should calculate 4 , 750.0 − 0136.2 = 46138 {\displaystyle 4,750.0-0136.2=46138} 4,750.0-0136.2=46138.

Drop the decimal point into your answer. The point in your answer should be aligned with the points in the numbers you were subtracting. For example, if you calculate 4 , 750.0 − 0136.2 = 46138 {\displaystyle 4,750.0-0136.2=46138} 4,750.0-0136.2=46138, you need to drop your decimal point in your answer so that it aligns with the other decimal points. So the answer is 4613.8.

Check your answer. In a problem like this, it is easy to make a mistake when aligning decimals or borrowing, so it never hurts to check your answer with a calculator.