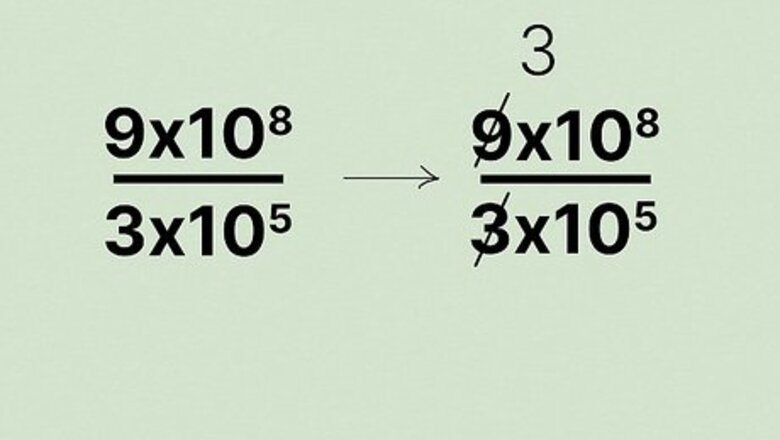
views
- Divide the whole numbers, then use the rule of exponents to divide the bases.
- If the new coefficient is a whole number, multiply the number by the new power of 10 to get your solution.
- If the new coefficient is not a whole number, convert it to scientific notation before multiplying it by the new power of 10.
Divide the coefficients.
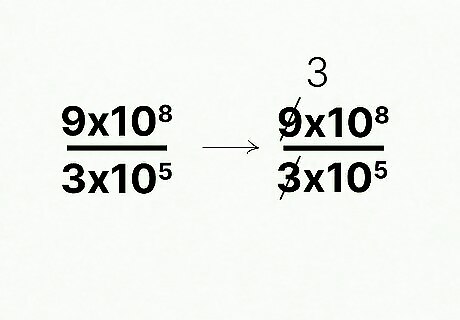
Start by dividing the whole numbers separately from the bases. This will give you your new coefficient in your scientific notation answer. For example: Let’s say the problem is ( 9 × 10 8 ) / ( 3 × 10 5 ) {\displaystyle (9\times 10^{8})/(3\times 10^{5})} {\displaystyle (9\times 10^{8})/(3\times 10^{5})} Our first step would be to divide the coefficients: 9 / 3 = 3 {\displaystyle 9/3=3} {\displaystyle 9/3=3}
Divide the bases.
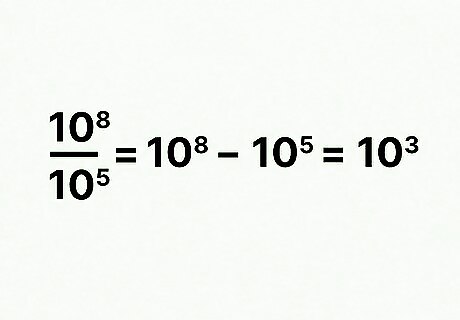
Use the rule of exponents to divide the bases separately. Now that you have your coefficient, it’s time to find the base. The rule of exponents tells us that to divide numbers with exponents, you just subtract them. To continue our example from above, dividing the bases would look like this: 10 8 / 10 5 {\displaystyle 10^{8}/10^{5}} {\displaystyle 10^{8}/10^{5}} Using the rule of exponents, we’d convert it to 10 8 − 10 5 = 10 3 {\displaystyle 10^{8}-10^{5}=10^{3}} {\displaystyle 10^{8}-10^{5}=10^{3}}, because 8 − 5 = 3 {\displaystyle 8-5=3} {\displaystyle 8-5=3}
Multiply whole numbers by the new power of 10.
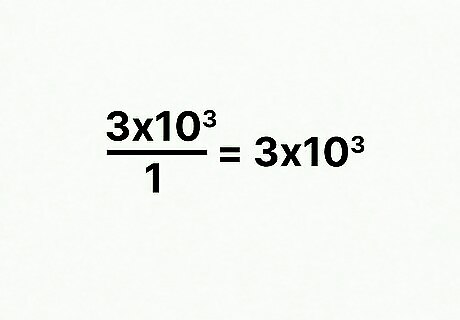
If your coefficient is a whole number, combine it with the base. Whole numbers are easy: all you have to do is take your coefficient that you found earlier and pair it with your new power of 10. From our example above, the answer would be 3 × 10 3 {\displaystyle 3\times 10^{3}} {\displaystyle 3\times 10^{3}}
Convert the coefficient to scientific notation if needed.
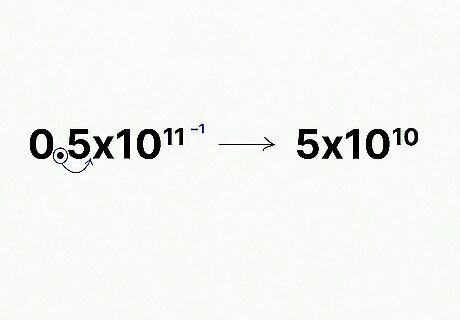
If your coefficient is not a whole number, convert it to scientific notation. Let’s say that when you divide your coefficient, the answer is a number that’s less than 1. In that case, there’s one more step you need to do: move the decimal point to the right, then decrease the exponent by the number of places that you move the decimal point. Let’s try a new problem: ( 2 × 10 3 ) / ( 4 × 10 − 8 ) {\displaystyle (2\times 10^{3})/(4\times 10^{-8})} {\displaystyle (2\times 10^{3})/(4\times 10^{-8})} ( 2 / 4 ) × ( 10 3 / 10 − 8 ) {\displaystyle (2/4)\times (10^{3}/10^{-8})} {\displaystyle (2/4)\times (10^{3}/10^{-8})} 0.5 × 10 11 {\displaystyle 0.5\times 10^{11}} {\displaystyle 0.5\times 10^{11}} Since the coefficient is less than 1, we now have to convert it to scientific notation: 0.5 = 5 × 10 − 1 {\displaystyle 0.5=5\times 10^{-1}} {\displaystyle 0.5=5\times 10^{-1}} Now we multiply the coefficient by the new power of 10 we solved for earlier: ( 5 × 10 − 1 ) × ( 10 11 ) {\displaystyle (5\times 10^{-1})\times (10^{11})} {\displaystyle (5\times 10^{-1})\times (10^{11})} The solution is 5 × 10 10 {\displaystyle 5\times 10^{10}} {\displaystyle 5\times 10^{10}}















Comments
0 comment